着色 Voronoi 图
·
问题:着色 Voronoi 图
我正在尝试为使用scipy.spatial.Voronoi创建的 Voronoi 图着色。这是我的代码:
import numpy as np
import matplotlib.pyplot as plt
from scipy.spatial import Voronoi, voronoi_plot_2d
# make up data points
points = np.random.rand(15,2)
# compute Voronoi tesselation
vor = Voronoi(points)
# plot
voronoi_plot_2d(vor)
# colorize
for region in vor.regions:
if not -1 in region:
polygon = [vor.vertices[i] for i in region]
plt.fill(*zip(*polygon))
plt.show()
结果图像:
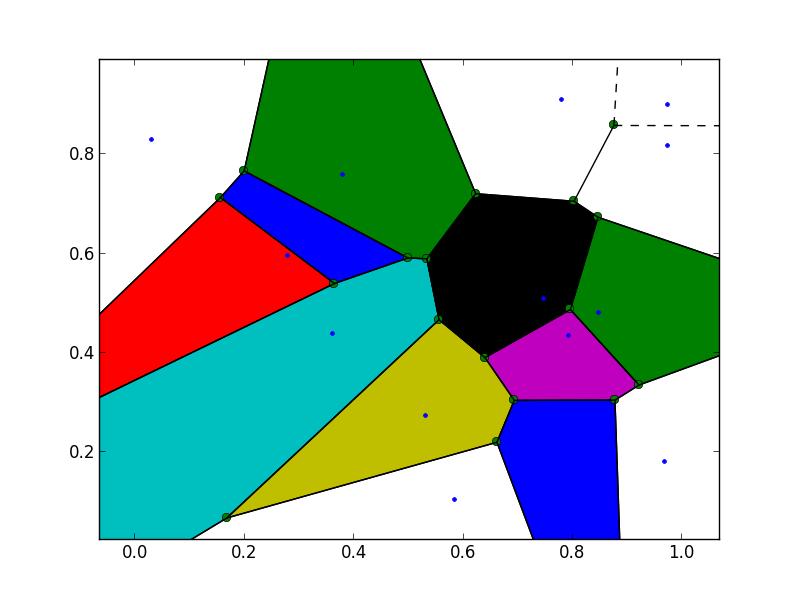
如您所见,图像边界处的一些 Voronoi 区域没有着色。这是因为这些区域的 Voronoi 顶点的一些索引设置为-1,即,对于 Voronoi 图之外的那些顶点。根据文档:
regions: (list of ints, shape (nregions, *)) 构成每个 Voronoi 区域的 Voronoi 顶点的索引。 -1 表示 Voronoi 图之外的顶点。
为了也为这些区域着色,我尝试从多边形中删除这些“外部”顶点,但这没有用。我想,我需要在图像区域的边界处填充一些点,但我似乎无法弄清楚如何合理地实现这一点。
任何人都可以帮忙吗?
解答
Voronoi 数据结构包含构造“无穷远点”位置所需的所有信息。 Qhull 还将它们简单地报告为-1索引,因此 Scipy 不会为您计算它们。
https://gist.github.com/pv/8036995
http://nbviewer.ipython.org/gist/pv/8037100
import numpy as np
import matplotlib.pyplot as plt
from scipy.spatial import Voronoi
def voronoi_finite_polygons_2d(vor, radius=None):
"""
Reconstruct infinite voronoi regions in a 2D diagram to finite
regions.
Parameters
----------
vor : Voronoi
Input diagram
radius : float, optional
Distance to 'points at infinity'.
Returns
-------
regions : list of tuples
Indices of vertices in each revised Voronoi regions.
vertices : list of tuples
Coordinates for revised Voronoi vertices. Same as coordinates
of input vertices, with 'points at infinity' appended to the
end.
"""
if vor.points.shape[1] != 2:
raise ValueError("Requires 2D input")
new_regions = []
new_vertices = vor.vertices.tolist()
center = vor.points.mean(axis=0)
if radius is None:
radius = vor.points.ptp().max()
# Construct a map containing all ridges for a given point
all_ridges = {}
for (p1, p2), (v1, v2) in zip(vor.ridge_points, vor.ridge_vertices):
all_ridges.setdefault(p1, []).append((p2, v1, v2))
all_ridges.setdefault(p2, []).append((p1, v1, v2))
# Reconstruct infinite regions
for p1, region in enumerate(vor.point_region):
vertices = vor.regions[region]
if all(v >= 0 for v in vertices):
# finite region
new_regions.append(vertices)
continue
# reconstruct a non-finite region
ridges = all_ridges[p1]
new_region = [v for v in vertices if v >= 0]
for p2, v1, v2 in ridges:
if v2 < 0:
v1, v2 = v2, v1
if v1 >= 0:
# finite ridge: already in the region
continue
# Compute the missing endpoint of an infinite ridge
t = vor.points[p2] - vor.points[p1] # tangent
t /= np.linalg.norm(t)
n = np.array([-t[1], t[0]]) # normal
midpoint = vor.points[[p1, p2]].mean(axis=0)
direction = np.sign(np.dot(midpoint - center, n)) * n
far_point = vor.vertices[v2] + direction * radius
new_region.append(len(new_vertices))
new_vertices.append(far_point.tolist())
# sort region counterclockwise
vs = np.asarray([new_vertices[v] for v in new_region])
c = vs.mean(axis=0)
angles = np.arctan2(vs[:,1] - c[1], vs[:,0] - c[0])
new_region = np.array(new_region)[np.argsort(angles)]
# finish
new_regions.append(new_region.tolist())
return new_regions, np.asarray(new_vertices)
# make up data points
np.random.seed(1234)
points = np.random.rand(15, 2)
# compute Voronoi tesselation
vor = Voronoi(points)
# plot
regions, vertices = voronoi_finite_polygons_2d(vor)
print "--"
print regions
print "--"
print vertices
# colorize
for region in regions:
polygon = vertices[region]
plt.fill(*zip(*polygon), alpha=0.4)
plt.plot(points[:,0], points[:,1], 'ko')
plt.xlim(vor.min_bound[0] - 0.1, vor.max_bound[0] + 0.1)
plt.ylim(vor.min_bound[1] - 0.1, vor.max_bound[1] + 0.1)
plt.show()
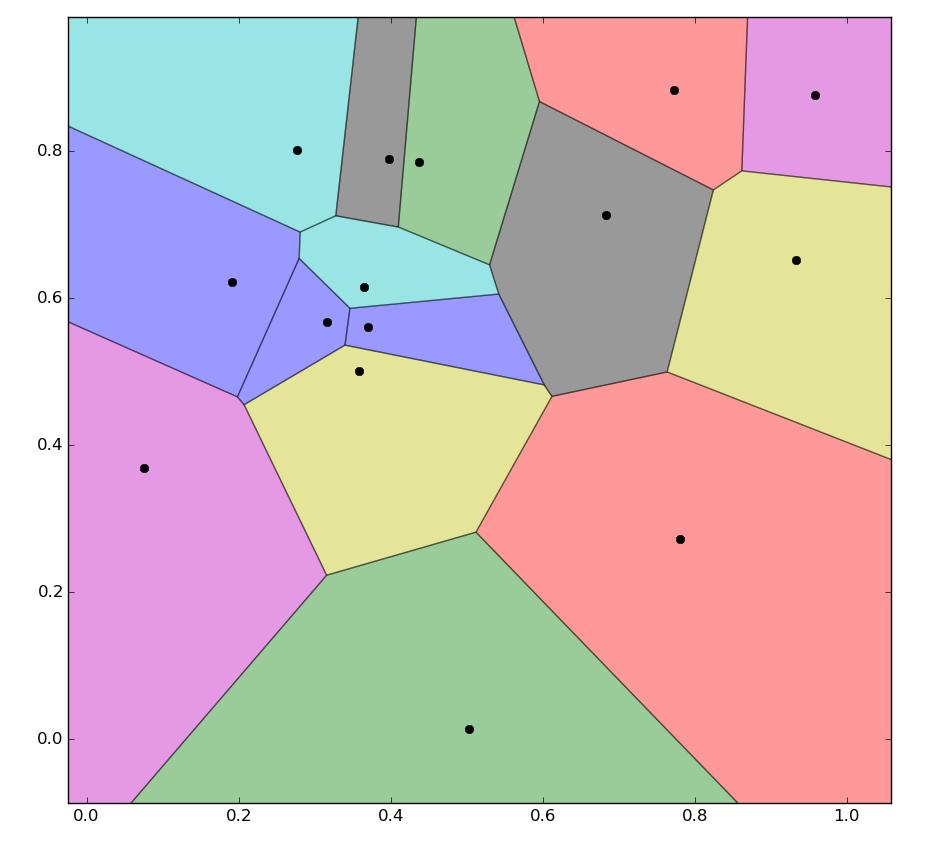
更多推荐
 已为社区贡献126450条内容
已为社区贡献126450条内容








所有评论(0)