统计学——线性回归决定系数R2
·
决定系数(coefficient ofdetermination),有的教材上翻译为判定系数,也称为拟合优度。
决定系数反应了y的波动有多少百分比能被x的波动所描述,即表征依变数Y的变异中有多少百分比,可由控制的自变数X来解释.
表达式:R2=SSR/SST=1-SSE/SST
其中:SST=SSR+SSE,SST(total sum of squares)为总平方和,SSR(regression sum of squares)为回归平方和,SSE(error sum of squares) 为残差平方和。
注:(不同书命名不同)
回归平方和:SSR(Sum of Squares forregression) = ESS (explained sum of squares)
残差平方和:SSE(Sum of Squares for Error) = RSS(residual sum of squares)
总离差平方和:SST(Sum of Squares fortotal) = TSS(total sum of squares)
SSE+SSR=SST RSS+ESS=TSS
意义:拟合优度越大,自变量对因变量的解释程度越高,自变量引起的变动占总变动的百分比高。观察点在回归直线附近越密集。
取值范围:0-1.
举例:
假设有10个点,如下图:
我们R来实现如何求线性方程和R2:
# 线性回归的方程
mylr = function(x,y){
plot(x,y)
x_mean = mean(x)
y_mean = mean(y)
xy_mean = mean(x*y)
xx_mean = mean(x*x)
yy_mean = mean(y*y)
m = (x_mean*y_mean - xy_mean)/(x_mean^2 - xx_mean)
b = y_mean - m*x_mean
f = m*x+b# 线性回归方程
lines(x,f)
sst = sum((y-y_mean)^2)
sse = sum((y-f)^2)
ssr = sum((f-y_mean)^2)
result = c(m,b,sst,sse,ssr)
names(result) = c('m','b','sst','sse','ssr')
return(result)
}
x = c(60,34,12,34,71,28,96,34,42,37)
y = c(301,169,47,178,365,126,491,157,202,184)
f = mylr(x,y)
f['m']
f['b']
f['sse']+f['ssr']
f['sst']
R2 = f['ssr']/f['sst']
最后方程为:f(x)=5.3x-15.5
R2为99.8,说明x对y的解释程度非常高。
推荐内容
阅读全文
AI总结
更多推荐
 已为社区贡献1条内容
已为社区贡献1条内容
相关推荐
查看更多
DeepSeek-V3-0324
DeepSeek最新推出DeepSeek-V3-0324版本,参数量从6710亿增加到6850亿,在数学推理、代码生成能力以及长上下文理解能力方面直线飙升。
Open-Sora
Open-Sora:为所有人实现高效视频制作
Python
All Algorithms implemented in Python
热门开源项目
活动日历
查看更多
直播时间 2025-04-07 14:51:20


樱花限定季|G-Star校园行&华中农业大学专场
直播时间 2025-03-26 14:30:09


开源工业物联实战!
直播时间 2025-03-25 14:30:17


Heygem.ai数字人超4000颗星火燎原!
直播时间 2025-03-13 18:32:35


全栈自研企业级AI平台:Java核心技术×私有化部署实战
直播时间 2025-03-11 18:35:18


从0到1:Go IoT 开发平台的架构演进与生态蓝图



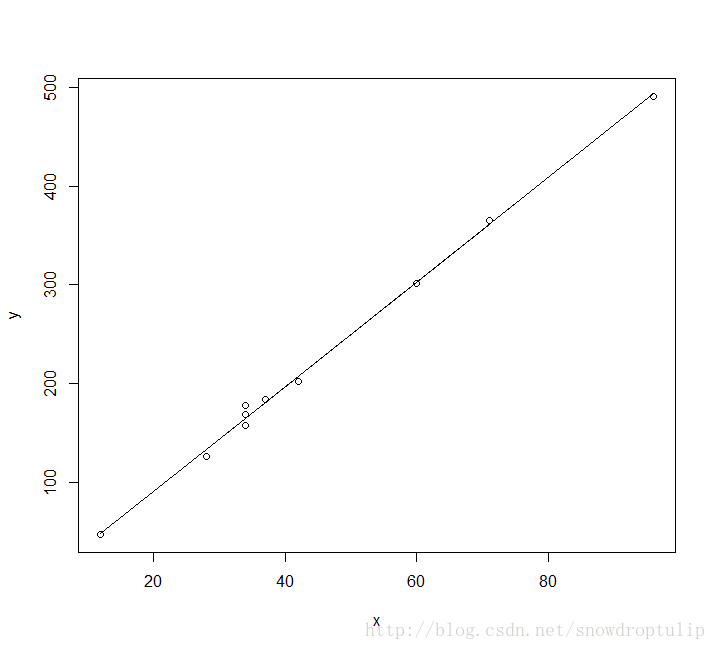










所有评论(0)